Doesn't seem possible, does it? Yet here it is.
Nerd level: max.
 www.theguardian.com
www.theguardian.com
Nerd level: max.
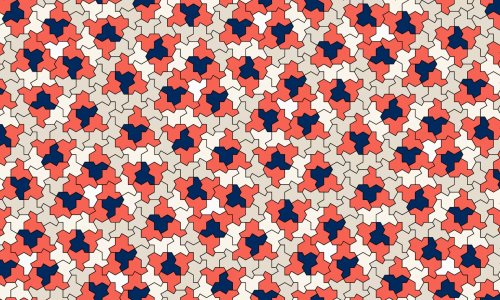
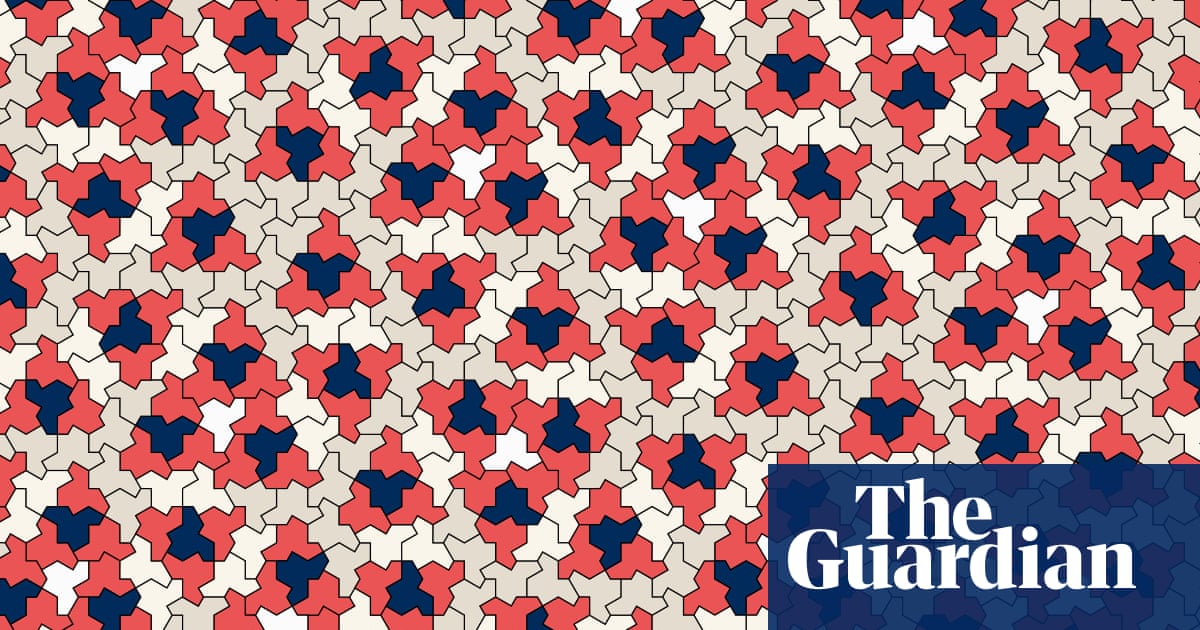
The conundrum: is there a shape that can be arranged in a tile formation, interlocking with itself ad infinitum, without the resulting pattern repeating over and over again?
In nature and on our bathroom walls, we typically see tile patterns that repeat in “a very predictable, regular way”, says Dr Craig Kaplan, an associate professor of computer science at the University of Waterloo in Ontario. What mathematicians were interested in were shapes that “guaranteed non-periodicity” – in other words, there was no way to tile them so that the overall pattern created a repeating grid.
Such a shape would be known as an aperiodic monotile, or “einstein” shape, meaning, in roughly translated German, “one shape” (and conveniently echoing the name of a certain theoretical physicist).


‘The miracle that disrupts order’: mathematicians invent new ‘einstein’ shape
Called ‘the hat’, the 13-sided shape can be arranged in a tile formation such that it never forms a repeating grid
